Nicolò Vignatavan - Linear equations and inequalities (ENG)
First
degree linear equations and inequalities: the fundamental differences of
representation on the Cartesian plane
A first degree equation is a polynomial
equation whose degree or maximum exponential numerical value of the unknown or
unknowns is 1, at the moment in which such polynomial, reduced to normal form,
or rather, after having added all the similar monomials that made it up, is set
equal to 0.
If the unknown exponential appears to be
of a numerical value greater than 1 in an equation reduced to normal form, then
such equation will be defined of a higher degree than the first.
The equations with one or more unknowns
or variables (if they result to be reduced to normal form and of first
degree) are called linear equations
because their representation on the Cartesian plane graphically corresponds to
straight lines; therefore linear geometric figures.
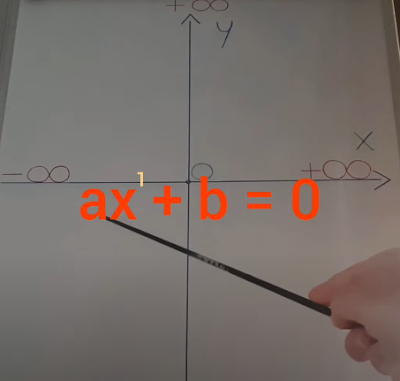
The normal form in which a one variable
(or unknown) first degree equation is reduced is the following: ax + b = 0,
which solved in x = -b / a; it is discussed in these terms:
if a is
different from 0 and b is different from 0, the equation allows a solution,
if a is
different from 0 and b is equal to 0, the equation is 0,
if a is
equal to 0 and b is not equal to 0, the equation is impossible,
if a is
equal to 0 and b is equal to 0, the equation is indeterminate.
Instead, a first degree inequality, therefore
linear, is a polynomial inequality with one or more unknowns or variables of
maximum exponential degree = 1, where
instead of the mathematical symbol "=" that testifies to a polynomial
equivalence ( typical of equations) the symbols ">",
"<", "> =", "<=" are introduced,
testifying a polynomial non-equivalence.
In one-dimensional space, therefore along
a line or an infinite axis, the result of a linear equation is always and only
a coordinate of a point graphically, while the result of a linear inequality is
a segment.
In two-dimensional space, therefore on a
classic Cartesian plane, the result of a linear equation is a straight line,
while the result of a linear inequality is an area.
The symbol of inequality “ > “or
“<” combined with the symbol of equality “=” in the algebraic result of the
inequation indicates how the point in question is included in the result and
therefore, projecting the latter on a one-dimensional line, it belongs to the
segment of the result of the inequation, while when projecting it on a
two-dimensional Cartesian plane, it belongs to the area of the result of the
inequality itself.
First degree equations reduced to
two-variable normal form will always represent incident lines with the
Cartesian axes.
y = +mx + q
y = -mx + q
First degree inequalities reduced to
two-variable normal form will always represent infinite areas for which their
side of origin will be incident with the Cartesian axes.
y> = mx + q
y <= mx + q
First degree equations reduced to
one-variable normal form will always represent orthogonal lines or lines
coincident to the Cartesian axes.
Orthogonal
y = q
x = q
with q other than 0
Coincident
y = 0
x = 0
First degree inequalities reduced to
one-variable normal form will always represent infinite areas for which their
side of origin will be orthogonal or coincident to the Cartesian axes.
Orthogonal
y> = q
y <= q
x> = q
x <= q
with q other than 0
Coincident
y> = 0
y <= 0
x> = 0
x <= 0
However, when considering the y-axis
starting from the explicit equation of the generic straight line, a different
graphical development is observed:
the explicit equation of a generic
straight line is: y = mx + q
As far as
“q” is concerned, it corresponds (for the ordinates) to the point of
intersection of the line with the y-axis. If the analyzed line is the y-axis,
we can see that it has no points of intersection with itself, but it is
coincident with itself. Therefore, considering the y-axis as a straight line
consisting of an infinite set of points, it will coincide with itself in
infinitive points and consequently, the value of “q" in its explicit
equation will be “infinite”.
As for the coefficient "m":
If "m" results to be the value
indicating the measurement of the vertical cathetus of the right triangle built
from q in the x direction and if the horizontal cathetus of this triangle
results to be a segment of length "1 unit", then:
If the straight line turns out to be
vertical, a case that we want to analyze now in order to study the value
of “m” in the equation of the Cartesian
y-axis , the following would happen:
the more the hypothetical straight line
that we want to coincide with the y-axis will increase its inclination and will
consequently get closer to the y-axis, the more its slope will have an ever
greater value and therefore, in the case of the y-axis or of a vertical
straight line which is by definition a straight line with a maximum slope, the
"m" slope will have the largest value that can be assumed; therefore
it will be infinite.
If the maximum inclination of a straight
line is given by the ratio between the infinite value of the vertical cathetus
of the triangle and the value 1 for the horizontal cathetus (although the
numerator is an infinite value, and so
its inclination, being maximum with respect to the x-axis, will generate a
vertical straight line), there will,
however, be an infinitesimal starting displacement with respect to the y-axis;
a unitary displacement that will not allow the perfect coincidence of the
explicit equation with m equal to infinity with the y-axis.
So, the following coincident line is
representative of the y-axis for the
equation y = oox + oo:
if m is equal to + infinity, it will
rotate by one infinitesimal clockwise with respect to the y-axis, and if m is
equal to - infinity, it will rotate by one infinitesimal counterclockwise with
respect to the y-axis.
Therefore, analyzing the redefinition of
the respective areas of influence generated by the linear inequality to a
variable corresponding to the y-axis on the Cartesian plane, considering the
axis of the ordinates starting from the explicit equation of the straight line,
the results will be as follows:
For y = oox + oo, understood as x = 0,
with respect to the x intervals
the inequality y> = + oox + oo will
generate the third quadrant of interval [-oo, 0-] and the second quadrant of
interval [-oo, o +], and the inequality y <= + oox + oo will generate the
first quadrant of interval [o +, + oo]
and the fourth quadrant of interval [o -, + oo].
the inequality y> = - oox + oo will
generate the first quadrant of interval [0 -, + oo] and the fourth quadrant of
interval [0 +, + oo] and the inequality y <= - oox + oo will generate the
third quadrant of interval [-oo, 0 +] and the second quadrant of interval [-oo,
0-].
Commenti
Posta un commento